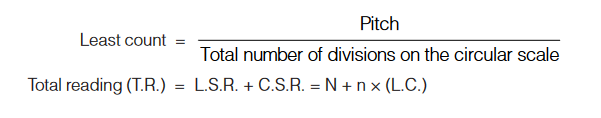Units and Measurement
FUNDAMENTAL FORCES IN NATURE
There are four fundamental forces in nature :
Gravitational Force
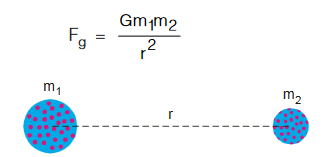
- Gravitational force is weakest force and conservative in nature.
- It is the force of mutual attraction between any two objects by virtue of their masses.
- It is a universal force and always attractive in nature.
- The gravitational force is appreciable only when at least one of the two bodies has a large mass.
Electromagnetic Force
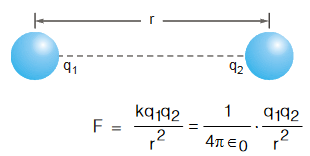
- Electromagnetic force is the force between charge particles.
- They are attractive as well as repulsive in nature.
- When charges are in motion, they produce magnetic field giving rise to a force on a moving charge.
- It is quite strong compared to gravity.
- For example, electric force between two protons is 10 36 times the gravitational force between them, for a certain distance.
Strong Nuclear Force
- It is independent of charge.
- The strong nuclear force is the strongest of all fundamental forces.
- Its range is extremely small of the order nuclear dimensions (10–15 m).
- It is responsible for the stability of nuclei.
Weak Nuclear Force
- The weak nuclear force is not as weak as the gravitational force but much weaker than strong nuclear force.
- The weak nuclear force appears only in certain nuclear processes β-decay of a nucleus.
- The range of weak nuclear force is exceedingly small of the order 10–36 m.
- Range of gravitational force > Range of electromagnetic force > Range of nuclear force.
- Strength of nuclear force > Strength of electromagnetic force > Strength of gravitational force

UNITS
| S.No. | Fundamental Physical Quantity | Units | Symbol |
|---|---|---|---|
| 1. | Length | metre | m |
| 2. | Mass | kilogram | kg |
| 3. | Time | second | s |
| 4. | Electric Current | ampere | A |
| 5. | Luminous Intensity | candela | cd |
| 6. | Temperature | kelvin | K |
| 7. | Amount of Substance | mole | mol |
| Supplementary fundamental quantities | Supplementary Units | ||
|---|---|---|---|
| Name | Symbol | Definition | |
| Plane angle | Radian | rad |
One radian is the angle subtended at the centre of a circle by an arc equal in length to the radius of the circle, i.e., dθ = ds/r |
| Solid angle | Steradian | sr |
One steradian is the solid angle subtended at the centre of a sphere, by that surface of the sphere, which is equal in area, to the square of radius of the sphere, i.e., dΩ = dA/r2 |
However, definition of units is under constant review and is changed from time to time. A body named “General conference on Height and Measures” holds the meeting to decide any changes in units.
Other International Systems are:
- The F.P.S. System is the British Engineering system of units. In this system unit of length is foot, of mass is pound and of time is second.
- The C.G.S. System is the Gaussian system. In this system units of length, mass and time are centimetre, gram and second respectively.
- The M.K.S. System in this system the units of length, mass and time are metre, kilogram and second respectively.
DIMENSIONS
Application of Dimensional Analysis
- In Conversion of Units from One System to Other
- To Check the Dimensional Correctness of a Given Physical Relation
- To Establish the Relation among Various Physical Quantities
- To Check the Dimensional Correctness of a Given Physical Relation
- To Establish the Relation among Various Physical Quantities
- To Find Dimensions of Physical Constants or Coefficients
SIGNIFICANT FIGURES
The rules for determining the number of significant figures are as follows :
- All the non-zero digits are significant.
- All the zeros between two non-zero digits are significant, no matter where the decimal point is
- If the number is less than 1, the zeros on the right of decimal point but to the left of 1st non-zero digit are not significant.
- All the zeros to the right of the last non-zero digit (trailing zeros) in a number without a decimal point are not significant, unless they come from experiment.
- The trailing zeros in a number with a decimal point are significant.
Significant Figure in Algebraic Operation
- In addition, or subtraction, the final result should retain as many decimal places as are there in the number with the least decimal place.
- In multiplication or division, the number of significant digits in the final result should be equal to the number of significant digits in the quantity, which has the minimum number of significant digits.
Rounding Off The Uncertain Digit (Least Significant Digit)
The least significant digit is rounded according the rules given below :- If the digit next to the least significant (Uncertain) digit is more than 5, the digit to be rounded is increased by 1.
- If the digit next to the one rounded is less than 5, the digit to be rounded is left unchanged.
- If the digit next to the one rounded is 5, then the digit to be rounded is increased by 1 if it is odd and left unchanged if it is even.
ERROR ANALYSIS IN EXPERIMENTS
(i) Mean of Observations: Suppose the observations of a physical quantity are X1, X2, X3 ….. Xn. Arithmetic mean of these observations Xmean is defined as :
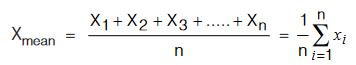 The arithmetic mean of these observations is taken as best possible value of the quantity under the given conditions of measurement.
The arithmetic mean of these observations is taken as best possible value of the quantity under the given conditions of measurement.
(ii) Absolute Error: The magnitude of the difference between the true value of the quantity and the individual measurement value is called the absolute error of the measurement.
(Arithmetic mean is taken as true value of number of observations).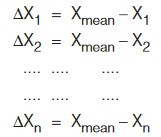 The absolute error may be positive or negative.
The absolute error may be positive or negative.
(iii) Absolute Mean Error: The average of the mod of errors is called the average or mean error.
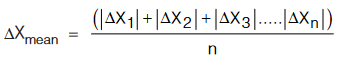
(iv) Relative Error: The relative error is the ratio of the mean absolute error ∆Xmean to the mean value Xmean of the quantity measured.
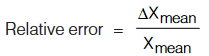 (v) Percentage Error: When the relative error is expressed in percent, it called the percentage error.
(v) Percentage Error: When the relative error is expressed in percent, it called the percentage error.

Combination of Errors
Errors in Sum or Difference Let, X = A ± BFurther, let ∆A is the absolute error in the measurement of A, ∆B the absolute error in the measurement of B and ∆X is the absolute error in the measurement of X.
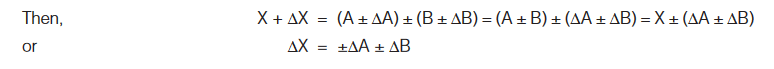 Errors in Product
Errors in Product
 Therefore, maximum fractional error in product of two (or more) quantities is equal to sum of fractional errors in the individual quantities.
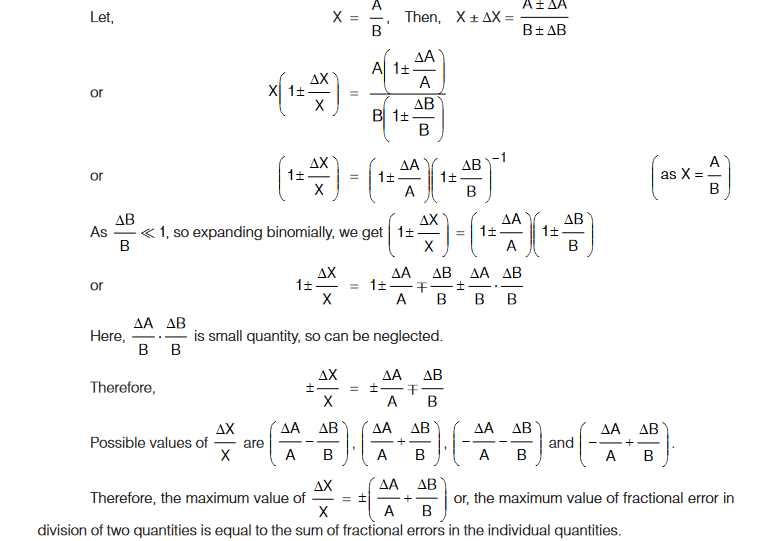
Errors in Division
Therefore, maximum fractional error in product of two (or more) quantities is equal to sum of fractional errors in the individual quantities.
Errors in Division
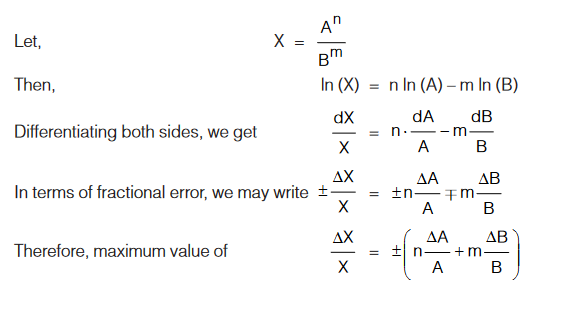
Errors in Quantity raised to Some Power
MEASURING INSTRUMENT
Vernier Callipers
Lease count = 1 M.S.D. – 1 V.S.D. Total reading, T.R. = M.S.R. + V.S.R. = N + n × (V.C.)Screw Gauge